Young children make strange errors of reasoning, but it isn’t because they are clueless. Studies of cognitive development suggest we’re partly to blame. We’re testing them the wrong way. In addition, kids are held back by an immature impulse control system — they are more likely to blurt out the first thing that comes to mind, even if they know it’s incorrect.

Around the world, people hold similar views about the timing of cognitive development: Young children aren’t capable of reason, and don’t make the shift to rationality until they are between 5 and 7 years old (Rogoff 1996). Are their beliefs accurate? Historically, there has been research on their side.
Jean Piaget’s landmark studies indicated that kids don’t grasp logic until they are approximately seven years old (Inhelder and Piaget 1958). And classic “theory of mind” experiments suggest that young children are poor psychologists. They act as if they don’t understand the perspectives of other people (Wimmer and Perner 1983; Perner and Rossler 2012).
But in recent decades, researchers have re-examined old assumptions and found reason for doubt.
Kids, they say, may be confused by the experimental procedures. They may be puzzled by the unnatural wording of the test questions, or distracted by too many details.
And there is another possibility:
All people, even highly-educated adults, experience misleading intuitions and cognitive biases. Getting the right answer depends on our ability to ignore these inner voices. Perhaps children say illogical things because they have trouble turning off their misleading intuitions.
Where’s the evidence? Consider first the weird things that young children say.
Three infamous failures of reasoning
1. Can children think clearly about sets?
Are there more red flowers in this picture, or more flowers?
An adult shows a child a bunch of flowers. Four are red, two are white.
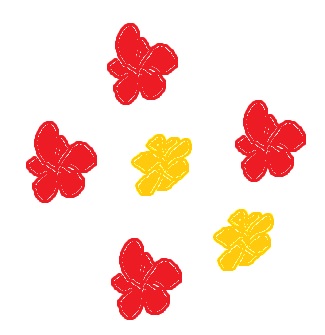
The adult asks, “Are there more red flowers or more flowers?“
Kids younger than six usually answer “There are more red flowers.”
2. Do children understand that different people can believe different things?
A child watches a puppet show about two characters — Maxi and his mother. The story goes like this:
- Maxi sees his mother put some chocolate in a blue cupboard.
- Maxi goes out to play.
- While Maxi is gone, his mother uses some of the chocolate to make a cake. Then she puts the remaining chocolate in a new location – a green cupboard.

At this point, the action pauses. The watching child should know that Maxi is ignorant about the relocation of the chocolate. He was out of the room when it happened.
So if Maxi wants chocolate, he’ll naturally look in the wrong place — the old location. The blue cupboard.
But does the child — whose seen everything happen — understand of all this? Does the child understand that Max holds a false belief?
To find out, an adult experimenter asks the child a question. He asks the child to anticipate what Maxi will do next.
“When Maxi comes back, where will Maxi look for the chocolate?”
Before the age of four, most kids say that Maxi will look for the chocolate in the new location, not in the blue cupboard. It’s as if children assume that their own knowledge is automatically shared by others.
3. Do kids understand the conservation principle — that you can’t increase or decrease a number of objects by merely moving them around?

A four- or five-year-old examines two identical rows of coins. She notes that each row contains the same number of coins.
But when an adult alters the top row–pushing together the coins so that the top row appears shorter–the child amends her assessment.
Now she says that the top row contains fewer coins.

Explaining the apparent irrationality of children
These outcomes have been replicated in populations around the world. What’s going on? Do the errors reflect limitations imposed by cognitive development? Or does a child’s performance depend on the ways we test her? Maybe it’s a combination of both.
It’s no surprise that children are sensitive to the social context of language–what linguists call “pragmatics.” When adults ask kids weird questions—as they often do in experiments designed to test cognitive development—kids may wonder why.
Misunderstandings about logic — or about the meaning of ambiguous questions?
Consider the flower task.
It’s from an experiment designed by Jean Piaget, and it’s supposed to test whether or not kids understand class inclusion or set theory, i.e., the idea that all the members of one set (red flowers) can belong to another, more inclusive set (flowers).

But the question about flowers—”Are there more red flowers, or more flowers?” is weird.
People don’t talk that way in everyday life. In fact, it’s likely that children have never been asked such a question.
So maybe, the child reasons, the adult isn’t speaking literally. Maybe the adult is really asking me to compare the red flowers with the white flowers.
Might this explain the error?
One study asked children about sets using different language:
Here is a bunch of grapes. There are green grapes and there are purple grapes, and this is the bunch. Who would have something more to eat, someone who ate the green grapes or someone who ate the bunch?
This is a more natural way of asking the question. And it seems to make a difference.
When kids were given this “natural language” version of the test, they did significantly better (Markman and Seibert 1976).
Numerous other studies have followed suit, finding ways to disambiguate the meaning behind class inclusion questions. When adults tweak the wording, kids are a lot less likely to make errors (Politzer 2016).
Moreover, researchers have demonstrated children’s competence with sets by devising entirely new tasks.
For instance, Usha Goswami and Sabina Pauen tested class inclusion reasoning by asking 4-and 5-year-olds to “create a family” by sorting through a group of mixed toys.
To succeed, kids needed to recognize one inclusive category (the species) and two subcategories (big and small animals of the same species).
Children managed it, and extended the idea to sort other objects, like blocks and ballooons (Goswami and Pauen 2005).
Theory of mind errors

Now let’s tackle the story about Maxi and his mother. That scenario is designed to test what psychologists call “theory of mind” skills — our ability to attribute thoughts, beliefs, and desires to other people.
Do young children assume that their own beliefs are shared universally by everyone? Do they believe that Maxi knows the true location of the chocolate, even though he was out of the room?
Or do children understand that Maxi has a mind of his own, and is capable of believing something different — something false?
As noted above, kids younger than four years tend to have trouble with the hidden chocolate problem. When asked where an ignorant character will look for the chocolate, the kids give the wrong answer. They claim that the character will look where the chocolate really is, not where the character should believe it to be.
This might indicate that two- and three-year-olds don’t attribute independent mental states to other people. A pretty disturbing thought!
But when researchers have measured false belief understanding by alternative methods, they’ve come up with compelling evidence that young children — even babies — really do know something about false beliefs (Onishi and Baillargeon 2005; Perner and Rossler 2012).
For example, consider this live action false belief scenario — one involving human actors.
- A child sees a woman place an object in a box.
- Then, while the woman is gone, the baby watches as the object is moved to another, hidden location.
- Finally, the woman returns and tries to recover the object.
What happens? Numerous experiments tell the same story:
If you don’t ask kids — but simply watch their eye movements — it’s clear that they anticipate that the woman will look for the item where she last left it, not in the new location.
Children as young as 18 months will even try to inform the woman of the change — pointing at the new hiding place (Scott and Baillargeon 2017; Knudsen and Liskowski 2012)!
So it’s clear that young children have an understanding of false beliefs. Why, then, do they routinely fail the traditional “Maxi” task?
Rose Scott and René Baillargeon (2017) think it’s because the traditional task is just too complicated. There is too much disparate information to juggle and process.
But perhaps it’s also about the wording of the question. In an amended version of the traditional false belief task, Michael Siegal and Katherine Beattie asked children,
“Where will Maxi look first for the chocolate?”
And with this wording, even three-year-olds tended to get the right answer most of the time.
Conservation errors
What about the last example—the one with the two rows of coins?

This is another task developed by Piaget, and it’s supposed to test a child’s understanding of conservation, the idea that quantities don’t change merely because we move things around.
It’s basic physics — and basic logic too. As David Elkind and Eva Schoenfeld (1972) noted, we can solve conservation tasks by using transitive inference:
- Row 1 has the same number of coins as does Row 2
- Row 1 has the same number of coins as does the squished-together version of Row 1
- Therefore, the squished-together version of Row one has the same number of coins as Row 2.
So are young children being hopelessly illogical when they fail at conservation tasks?
Once again, we need to consider the social context.
Imagine you’re the child being tested. You’ve just told the adult that both rows contain the same number of coins.
Next, the adult takes the coins in the first row and changes the spacing between them. Then she asks you the same question again—are there “more, less, or the same in each row?”
What are you to think?
Ordinarily, you’d assume that the number hasn’t changed. But why would this authority figure ask such an obvious question?
Maybe–for some unknowable reason–she wants or expects you to change your original answer. So you do.
Could this explain why so many young children have failed the conservation task? It might explain part of the phenomenon.
James McGarrigle and Margaret Donaldson gave the classic conservation task a tweak. After the child inspected the two rows and acknowledged that they were equivalent, the experiment was “interrupted” by a “naughty” teddy bear who shifted the coins. The adult scolded the bear and asked the question again.
In this scenario, even four-year-olds tended to get the right answer (McGarricle and Donaldson 1975).
These results have been questioned by some researchers. Maybe the teddy bear distracted kids so much that they didn’t bother to rethink their answers.
And if you look at this demonstration video on YouTube (which shows several standard Piagetian conservation tasks), perhaps you can see why some people don’t believe that kids are in conflict.
I’m not an expert in body language. But to my untrained eye, these girls seem pretty sure of themselves.
On the other hand, they also seem very eager to please! Do they really believe the quantities have changed? Or are they convinced that the woman wants them to change their answers?
Maybe the answer is that kids shut off their critical thinking skills when faced with the social demands of the situation. Other experiments confirm that young children tend to investigate less when they are in the presence of an authoritative adult (Bonawitz et al 2011; Buchsbaum et al 2011).
I suspect that pragmatics and other social factors are influencing the way children perform Piagetian tasks.
So does social context account for all the errors?
No. As noted above, Rose Scott and René Baillargeon think there’s more going on with false belief task errors, and that’s likely true for other mistakes as well.
For example, all of the tasks we’ve discussed tap working memory, and working memory increases throughout the course of cognitive development. Young children are at a disadvantage.
Moreover, experience helps. A study of first graders suggests that kids benefit when they are explicitly trained to solve classification problems (Pasnak et al 2006)—a finding that’s consistent with the notion that logic and critical thinking must be taught.
But perhaps the most important factor isn’t working memory, or even a child’s prior understanding of the world. Instead, it’s a form of self-restraint: An individual’s ability to ignore easy — but misleading — intuitions.
People of all ages use simple heuristics, or rules-of-thumb, to solve everyday problems. It’s a mode of “fast thinking” that rewards us with quick, intuitive answers (Kahneman 2011). But sometimes it leads us astray. For instance, consider this heuristic:
“If there is a row of objects, use the length of that row to estimate quantity.”
This is usually a good rule-of-thumb, which is why people use it when deciding which line to stand in at the supermarket. But in the conservation experiment with the coins, the rule backfires. To get the right answer, you must selectively ignore the “length-equals-number” heuristic, and that requires conscious attention or inhibitory control.
Adults do it with effort. But young children have particular trouble (Houdé and Bourst 2014).
It’s harder for kids to override their intuitions
Do kids make logical errors because they can’t turn off their “fast thinking,” knee-jerk reactions? Olivier Houdé and his colleagues have championed the idea, and it explains a lot.
For example, consider the Stroop effect, which shows that people take longer to answer questions when those questions contain distracting elements.
Even when you know that the distractions are irrelevant, some part of your mind is captivated by them. Too see what I mean, try answering quickly:
Which of these animals is larger in real life?

Because the wolf looks larger, part of your mind wants to answer “wolf.” To get the right answer, you must inhibit that impulse, which takes extra effort and time.
Kids are slower at these sorts of tasks, and brain research suggests why. For kids, the brain activity associated with the incorrect response (e.g., the rabbit) is stronger and longer-lasting than it is for adults (Szucs et al 2009).
Moreover, there is less activity in key areas of the prefrontal cortex, brain regions associated with inhibitory control (Houdé and Borst 2015; Borst et al 2013). These areas are “under construction” in young children, and continue to develop throughout later childhood and adolescence (Casey et al 2005).
Kids don’t show big improvements in Stroop-like tasks until they are around 8 years old (Ikeda et al 2014).
So kids really do know that elephants are bigger than wolves, and they are capable of understanding that 5 coins don’t become 6 coins merely because we move them around.
But they have more trouble inhibiting the wrong answer. Their internal censor–the executive function that stops us from blurting out silly things–isn’t as powerful.
That’s an important developmental constraint on reasoning, but it doesn’t mean kids are fundamentally irrational or illogical.
Indeed, as Houdé and Gregoire Borst point out, young babies routinely pass tests of number conservation in the laboratory. They seem to understand that moving objects around can’t change their number, and they don’t have to inhibit the “length-equals-number” heuristic. They haven’t learned it yet!
We acquire intuitions and rules-of-thumb throughout our lives, and frequently have to chose between trusting these heuristics or taking a more effortful, careful approach to problem-solving. Adults, like children, can get it wrong, and we all benefit from learning to scrutinize the easy answers.
More information about cognitive development during early childhood
To read more about the cognitive development of very young children, see these other Parenting Science articles:
- Talking to babies: How friendly eye contact can make infants tune in – and mirror your brain waves
- Babies can tell when you’re stressed
- Can babies sign before they speak?
- When do babies say their first words?
- What babies know about numbers
- At what age do kids begin telling lies?
For thoughts on the ways that adults negatively affect cognitive performance in children, see my evidence-based essay about children’s media.
For tips on encouraging kids to learn from mistakes, see my article, “Correcting behavior: the magic words.”
For tips on supporting the development of rationality and inhibitory control, see these articles about teaching critical thinking and self-discipline.
References: Preschool cognitive development
Birch SAJ and Bloom P. 2007. The curse of knowledge in reasoning about false beliefs. Psychological Science 18(5): 382-386.
Borst G, Aïte A, and Houdé O. 2015. Inhibition of misleading heuristics as a core mechanism for typical cognitive development: evidence from behavioural and brain-imaging studies. Dev Med Child Neurol. 57 Suppl 2:21-5.
Borst G, Poirel N, Pineau A, Cassotti M, Houdé O. 2013. Inhibitory control efficiency in a Piaget-like class-inclusion task in school-age children and adults: a developmental negative priming study. Dev Psychol. 49(7):1366-74.
Bonawitz E, Shafto P, Gweon H, Goodman ND, Spelke E and Shultz L. 2011. The double-edged sword of pedagogy: Instruction limits spontaneous exploration and discovery. Cognition 120(3): 322-330.
Buchsbaum B, Gopnik A, Griffiths TL, and Shafto P. 2011. Children’s imitation of causal action sequences is influenced by statistical and pedagogical evidence. Cognition 120(3): 331-340.
Casey B, Tottenham N, Liston N, and Durston S. 2005. Imaging the developing brain. Trends Cogn Neuro 9:104-110.
Elkind D and Schoenfeld E. 1972. Identity and equivalence conservation at two age levels. Developmental Psychology 6: 529-533.
Goswami U and Pauen S. 2005. The effects of a “family” analogy on class inclusion reasoning by young children. Swiss Journal of Psychology, 64, 115-124.
Houdé O and Borst G. 2015. Evidence for an inhibitory-control theory of reasoning in the brain. Front Hum Neurosci 9:148.
Houdé O and Borst G. 2014. Measuring inhibitory control in children and adults: brain imaging and mental chronometry. Front Psychol. 5:616.
Ikeda Y, Okuzumi H, Kokubun M. 2015. Stroop-like interference in the real animal size test and the pictorial animal size test in 5- to 12-year-old children and young adults. Appl Neuropsychol Child. 3(2):115-25
Inhelder B and Piaget J. 1958. The Growth of Logical Thinking from Childhood to Adolescence. New York: Basic Books.
Knudsen B and Liszkowski U. 2012 Eighteen-and 24-month-old infants correct others in anticipation of action mistakes. Dev. Sci. 15: 113-122.
Markman E and Seibert J. 1976. Classes and collections: internal organization and resulting holistic properties. Cognitive Psychology 8: 561-577.
McGarrigle J and Donaldson M. 1975. Conservation accidents. Cognition 3: 341-350.
Moore C and Frye D. 1986. The effect of experimenter’s intention on the child’s understanding of conservation. Cognition. 22(3):283-98.
Onishi KH and Baillargeon R. 2005. Do 15-month-old infants understand false beliefs? Science 308: 255-258.
Perner J and Roessler J. 2012. From infants’ to children’s appreciation of belief. Trends Cogn Sci. 16(10):519-25.
Politzer G. 2016. The class inclusion question: a case study in applying pragmatics to the experimental study of cognition. Springerplus. 5(1):1133
Priewasser B, Roessler J, and Perner J. 2012. Competition as rational action: Why young children cannot appreciate competitive games. J Exp Child Psychol. Nov 2012 doi: 10.1016/j.jecp.2012.10.008. [Epub ahead of print].
Rogoff B. 1996. Developmental transitions in children’s participation in sociocultural activities. In A. Smameroff and M. M. Haith (eds): The five to seven year shift: The age of reason and responsibility. University of Chicago.
Scott RM and Baillargeon R. 2017. Early False-Belief Understanding. Trends Cogn Sci. 21(4):237-249.
Siegel M and Beattie D. 1991. Where to look first for children’s knowledge of false belief. Cognition 38: 1-12.
Stroop JR. 1935. Studies of interference in serial verbal reactions. J. Exp. Psychol. 18:643-662.
Szücs D, Soltész F, Bryce D and Whitebread, D. 2009. Real-time tracking of motor response activation and response competition in a Stroop task in young children: A lateralized readiness potential study, Journal of Cognitive Neuroscience 21(11): 2195-2206.
Wimmer H and Perner J. 1983. Beliefs about beliefs: Representation and constraining function of wrong beliefs in young children’s understanding of deception. Cognition 13 (1): 103–128.
Content last modified 7/2019
image of teacher stacking cups with child by istock / Rawpixel
images of coins, sock puppet, and flowers by Parenting Science
image of large wolf and small elephant resized from image by istock / MacroVector
image of toddler girl ©iStockphoto.com/RonTech2000